Journal Club for Nov 2024: Manufacturing, Testing, and Actuation of Origami-Based Metamaterials

Diego Misseroni

Laboratory for the Design of Reconfigurable Metamaterials & Structures, Department of Civil, Environmental and Mechanical Engineering, University of Trento, Italy

Introduction

Origami-based metamaterials are highly deployable systems that require precise consideration of manufacturing techniques and experimental setups to accurately assess their mechanical behavior and validate theoretical models. A significant challenge lies in bridging the gap between idealized assumptions, often used in theoretical studies, and the complexities of real-world testing. For instance, many models assume that panels are infinitely rigid while having zero thickness, an assumption that simplifies analysis but is nearly impossible to achieve in practice. Consequently, ensuring that experimental setups account for material properties, geometric imperfections, and realistic boundary conditions becomes essential for obtaining meaningful results that are repeatable and align with theoretical predictions. Careful calibration of these factors is critical for advancing the development and practical application of these advanced materials [1]. In the following sections, we will: (i) explore the primary manufacturing techniques employed to create origami metamaterials, (ii) analyze the experimental setups used for testing them, and (iii) provide an overview of the current limitations in this field and potential future directions for research and development.

Manufacturing methods

The selection of appropriate manufacturing methods for creating origami metamaterials is crucial to ensure reliable and accurate samples for testing. There is no single universal method; instead, the choice of manufacturing technique depends largely on the type of experiments being. Such a choice is influenced by factors like the base materials used, the design of the structure, the scale, and the type of mechanical testing required. Each method must be carefully matched to the specific needs of the experiment to ensure accurate results.

Laser cutter

The most straightforward method for creating origami metamaterials involves the use of a laser cutter (Fig. 1A). In this approach, the metamaterials are typically paper based. The tessellation patterns are generated by cutting strips or shapes directly from a 2D sheet of paper. Creases, which enable folding, are either formed by etching the surface of the paper or by making dashed cuts along the desired fold lines. While paper is the most common material, polyester film (e.g., Grafix Drafting Film) and composite film (e.g., Durilla Durable Premium Ice Card Stock) [2, 3, 4] can also be used to create more durable samples, offering a range of mechanical properties depending on the material choice and the precision of the cuts.

CNC (computer numerical control) milling

Another method for manufacturing tessellations is by using a CNC milling machine (Fig. 1B). In this process, tessellations (for developable patterns) or individual strips (for non-developable patterns) are cut from a thin sheet of plastic material, such as polypropylene or polycarbonate, using a cutting tool. The creases are created by engraving the sheet to a controlled depth, typically using a ball-end tool. This method allows for high precision and produces reliable prototypes, especially when investigating behaviors like multistability or auxetic properties [5]. However, a key limitation is that the samples cannot be too small, and for non-developable patterns, the tessellation requires post-assembly by gluing several strips or basic elements together.

3D printing

A more advanced method for creating origami tessellations is 3D printing (Fig. 1C). In this process, designs are first modeled using 3D CAD software, which generates the source file needed to operate the 3D printer. This approach enables the fabrication of tessellations using a range of materials, such as polymers and metals [6, 7]. Additionally, by incorporating active resins, 4D printing becomes possible, allowing for the creation of adaptive or self-folding origami structures [8, 9, 10]. Although this method offers significant advantages, including material versatility and the ability to produce complex designs, it also comes with certain technological limitations. These include challenges related to the viscosity of the printing materials, the degradation of printed parts over time, and difficulties in achieving precise miniaturization at smaller scales.

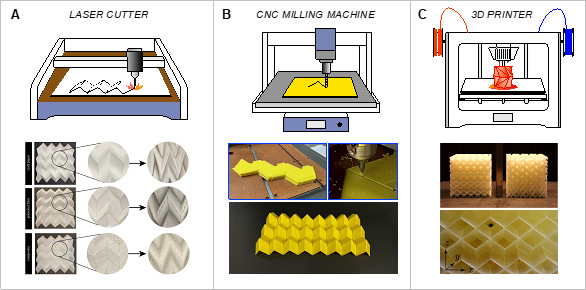
Figure 1: Main manufacturing methods to create origami-based metamaterials: (A) Laser cutting [2], (B) CNC milling machining [11], and (C) 3D printing [12]. 

Testing & Actuation

In-Plane Poisson ratio experiments

Proper testing of origami tessellations requires a carefully designed testing setup. Since these samples are highly deployable, performing tests using standard methods—such as clamping the two ends of the sample to a loading frame—can result in non-uniform transverse deformation. The constraints at the ends prevent the sample from contracting uniformly, causing a “dog-bone” shape that leads to inconsistent transverse contraction measurements and unreliable experimental results.

To address this issue, a specialized gripping system has been conceived, designed, and fabricated, as shown in (Fig. 2A) [11]. This system allows the sample to deform freely in the transverse direction while a longitudinal deformation is applied. The gripping system consists of multiple sliders placed within a rail. These sliders can move freely along the rail, allowing the tessellation itself to control the position of the sliders during the folding and unfolding process. This ensures uniform transverse deformation, effectively replicating periodic boundary conditions during the experiment and yielding more accurate and reliable results.

Kresling-based structures

Kresling cells are known for their chiral effect, where axial displacement and twisting are coupled. Typically, experiments on these systems are conducted on arrays with an even number of cells, arranged with opposite chirality to cancel out any relative rotation between the end surfaces. However, in more complex experiments—such as those involving arrays with an odd or even number of cells without constraints on the chiral arrangement—specialized setups are required to decouple the two degrees of freedom (axial displacement and twist). In these cases, two distinct setups are needed. The first setup should allow the sample to freely rotate while applying an axial displacement (Fig. 2B). The second setup should permit free axial displacement while a controlled rotation is applied to the sample (Fig. 2C). These configurations ensure that the interaction between axial and rotational motions is properly isolated, allowing for precise measurement of each degree of freedom during the experiments [13].

Figure 2: (A) Setup for In-plane Poisson’s ratio experiments [11], (B) and (C) Setups for experiments on Kresling array structures [13].

Fluidic actuated origami 

Origami folding can be controlled using fluidic actuation, where air pressure is used to manipulate the structure [6]. For this approach to work, the origami structures must be completely airtight to prevent any air leakage. The system is tethered, meaning that the structure must be connected to one or more tubes linked to an air supply system. This system regulates both air pressure and flow rate, allowing precise control of the folding and unfolding process. The effectiveness of this method relies on maintaining the airtight integrity of the origami structure and ensuring proper control over the air supply.

Magnetic actuated origami

Origami structures can also be actuated using magnetic fields, offering the key advantage of remote, tetherless control [14]. To achieve this, magnetic components are integrated into the origami design and magnetized beforehand. This method is especially valuable in situations where tethered control is impractical or cumbersome. For instance, magnetic actuation is ideal for medical applications, such as targeted drug delivery, where precise movement of the structure is needed without physical connections. It is also highly effective in soft robotics, particularly for controlling the omnidirectional motion of robotic arms or origami-based crawlers, which require flexibility and adaptability in their movements [15]. The ability to control these systems remotely significantly increases the versatility and effectiveness of magnetic actuation, making it suitable for a wide range of applications.

Stimuli responsive origami

The emergence of 4D printing has enabled the use of stimuli-responsive materials to control the shape-change of origami structures. These materials can respond to external stimuli, such as light, heat, or humidity (hygroscopic control), causing the structure to fold or unfold in a controlled manner. This technique is particularly promising in the development of self-folding origami, where the structure autonomously transforms in response to environmental changes. The ability to design materials that adapt over time adds a dynamic dimension to traditional origami, opening up new possibilities for applications in fields such as robotics, biomedical devices, and deployable structures [9].

Limitations and Outlook

Although several manufacturing techniques exist for creating origami-based systems, each has inherent limitations that prevent a single method from being universally suitable. For example, while 3D printing is often viewed as a revolutionary tool that can prototype nearly anything, using these prototypes for quantitative experiments requires careful consideration and attention to detail.

A notable issue with 3D printing, especially when using photoresins, is the degradation of mechanical properties over time. Photoresins are sensitive to light exposure, leading to significant changes in their performance. For instance, samples can exhibit a reduction in Young’s modulus of over 15% after just three months of light exposure, reaching up to 19% after six months. This weakening is even more pronounced in systems subjected to cyclic loading. For example, in multimaterial Kresling structures, the load-bearing capacity can drop by an average of approximately 30% and 50% after one and three loading cycles, respectively [16].  This highlights a critical flaw in relying on photoresin-based 3D prints for long-term or repeated-use applications without accounting for this degradation.

Miniaturization of origami structures is another significant challenge. While 3D printing has enabled the creation of complex forms at various scales, the precision required to create functional multistable Kresling systems at the microscale is extremely difficult with current technologies. Manufacturing tolerances, material behavior, and the resolution limits of 3D printers make it hard to achieve the delicate balance needed for microscale stability and actuation [17].

At the opposite end of the spectrum, scaling origami systems to macroscale presents its own difficulties. Large-scale origami structures often face issues related to actuation and material thickness. As the size increases, the thickness can hinder proper folding and unfolding, while the force needed for actuation becomes greater. This added complexity makes it more difficult to design reliable and efficient actuation mechanisms. Material limitations are also critical at larger scales; flexible materials that work well for smaller designs may not hold up under increased loads, resulting in issues like buckling or permanent deformation. Furthermore, the intricate folding patterns that function effectively on a small scale can become overly complicated when scaled up, complicating the manufacturing process and making it hard to ensure that the final structure performs as intended. 

References

[1] D. Misseroni, P. Phanisri P, K. Liu, B. Kresling, Y. Chen, C. Daraio and G. H. Paulino, “Origami engineering,” Nature Reviews Methods Primers, p. 40, 2024. 

[2] K. Liu, L. Novelino, P. Gardoni and G. Paulino, “Big influence of small random imperfections in origami-based metamaterials,” Proceedings of the Royal Society A, vol. 476, no. 2241, p. 20200236, 2020. 

[3] A. Jamalimehr, M. Morad, A. Abdolhamid and D. Pasini, “Rigidly flat-foldable class of lockable origami-inspired metamaterials with topological stiff states,” Nature communications, vol. 13, no. 1, p. 1816, 2022. 

[4] Y. Xia, N. Kidambi, E. Filipov and K.-W. Wang, “Deployment dynamics of Miura origami sheets,” Journal of Computational and Nonlinear Dynamics , vol. 17, no. 7, p. 071005, 2022. 

[5] K. Liu, P. P. Pratapa, D. Misseroni, T. Tachi and G. Paulino, “Triclinic metamaterials by tristable origami with reprogrammable frustration,” Advanced Materials, vol. 34, no. 43, p. 2107998, 2022. 

[6] D. Melancon, B. Gorissen, C. J. García-Mora, C. Hoberman and K. Bertoldi, “Multistable inflatable origami structures at the metre scale,” Nature, vol. 592, no. 7855, pp. 545-550, 2021. 

[7] Z. Zeang, X. Kuang, J. Wu, Q. Zhang,, G. H. Paulino, J. Qi and D. Fang, “3D printing of complex origami assemblages for reconfigurable structures,” Soft Matter, vol. 14, no. 39, pp. 8051-8059, 2018. 

[8] A. Kotikian, C. McMahan, E. C. Davidson, J. M. Muhammad, R. D. Weeks, C. Daraio and J. A. Lewis, “Untethered soft robotic matter with passive control of shape morphing and propulsion,” Science robotics, vol. 4, no. 33, p. eaax7044, 2019. 

[9] X. Xia, C. Spadaccini and J. R. Greer, “Responsive materials architected in space and time,” Nature Reviews Materials, vol. 7, pp. 683-701, 2022. 

[10] Z. Fang, H. Song, Y. Zhang, J. Binjie, J. Wu, Q. Zhao and T. Xie, “Modular 4D printing via interfacial welding of digital light-controllable dynamic covalent polymer networks,” Matter, vol. 2, no. 5, pp. 1187-1197., 2020. 

[11] D. Misseroni, P. P. Pratapa, K. Liu and G. H. Paulino, “Experimental realization of tunable Poisson’s ratio in deployable origami metamaterials,” Extreme Mechanics Letters, vol. 53, p. 101685, 2022. 

[12] K. C. Cheung, T. Tachi, S. Calisch and K. Miura, Origami interleaved tube cellular materials, vol. 23, Smart Mater. Struct., 2014, p. 094012.

[13] S. Zang, D. Misseroni, T. Zhao and G. H. Paulino, “Kresling origami mechanics explained: Experiments and theory,” Journal of the Mechanics and Physics of Solids, vol. 188, p. 105630, 2024. 

[14] L. Novelino, Z. Qiji, W. Shuai and G. Paulino, “Untethered control of functional origami microrobots with distributed actuation,” Proccedings of the National Academy of Sciences, vol. 117, no. 39, pp. 24096-24101, 2020. 

[15] S. Wu, Q. Ze, J. Dai, N. Udipi, G. H. Paulino and R. Zhao, “Stretchable origami robotic arm with omnidirectional bending and twisting,” Proceedings of the National Academy of Sciences , vol. 118, no. 36, p. e2110023118, 2021. 

[16] S. Mora, N. M. Pugno and D. Misseroni, “Impact of Crease Geometry and Viscosity on the Energy Landscape,” 2024. [Online]. Available: arXiv.

[17] S. Mora, N. M. Pugno and D. Misseroni, “3D printed architected lattice structures by material jetting,” Materials Today, vol. 59, pp. 107-132, 2022.